Have you ever needed to send an email and you have no idea how to start? That’s how some of our students feel when we give them a problem or problems that they may have no idea how to approach!
Enter worked examples!
Worked examples are step-by-step solutions or partial solutions to mathematical problems. Each step is clearly explained and visible for students to see.

Benefits of Using Worked Examples
Findings have shown that worked examples:
✅Show a model of problem-solving techniques and strategies,
✅Break down complex problems into smaller, more manageable steps,
✅Help students learn about specific problem-solving strategies that can be applied to other problems in the future,
✅Help students focus on understanding a concept or strategy without the cognitive load of figuring out all of the steps. (Let’s keep the main thing the main thing!)
Types of Worked Out Examples
There are a lot of different types of worked examples, each with their own benefits for students. The titles are self-explanatory but helpful to learn more about.
Fading Examples
Fading examples provide scaffolded fading support for students to solve problems more successfully. As students progress, the problems become less worked out with more steps for them to do. Students are more successful when steps are faded from the end than the beginning. When possible, this strategy can be very successful when the fading is specific to student needs.
Benefits of this style of worked examples
Better performance on hard problems
Less time is needed to achieve the same level of performance
Less unproductive moments of struggle
Prompting Self Explanation
In this style of worked example, you are asking students to explain information to themselves. Prompting self-explanation helps kids integrate prior knowledge with new information and forces them to make the new learning explicit.
In the math setting, having students explain what was done/why certain steps work help
Increase the likelihood of students being able to pick out relevant information
Helps in understanding underlying concepts in problems and the ability to carry out the steps
Increases declarative knowledge and conceptual knowledge and transfer learned content knowledge
Incorrect Worked Examples
Incorrect worked examples can help show common mistakes and discuss why certain steps don’t work.
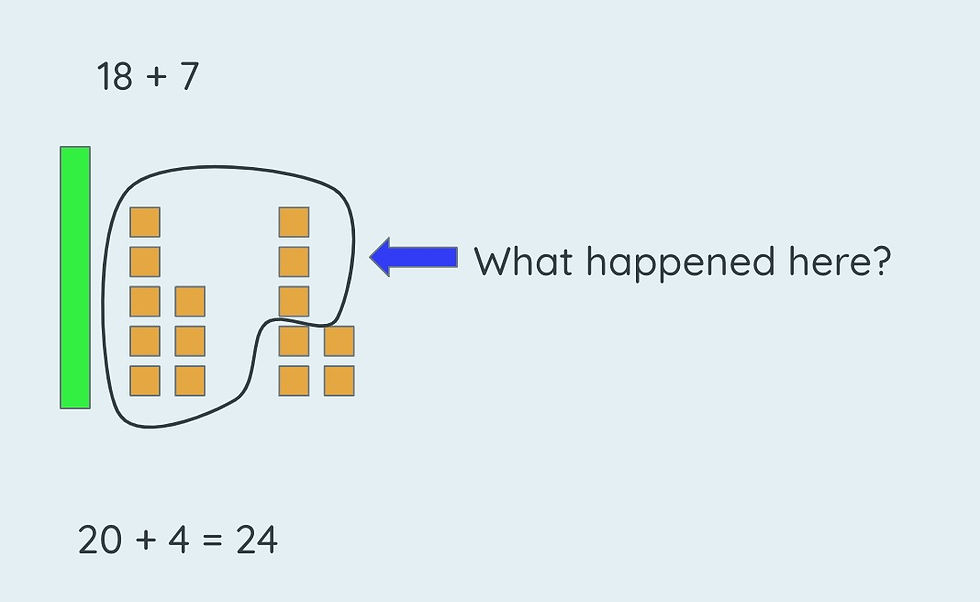
There can be some hesitation with showing students something incorrect, and you have to make it clear that the example you are showing is wrong and that together you are discussing why it doesn't work so that in the future they can avoid making the same mistake.
Using this type of worked example
Ideally shows common mistakes
Thought to help students figure out why steps are not working
Once aware of the mistake, increasing the likelihood they will not make the same mistake
Promotes the learning of correct concepts and procedures by contrast, reducing misconceptions about the content that may still be present when showing only correct examples
Similar to the previous one, explaining why something doesn’t work helps conceptual understanding
Works for both high and low-prior knowledge students.
Fine-tunes problem-solving strategies
Comparing Multiple Examples
Comparing two examples can help students make connections between different strategies and move them to more efficient strategies or develop flexibility enough to be able to choose the best strategy for a problem.
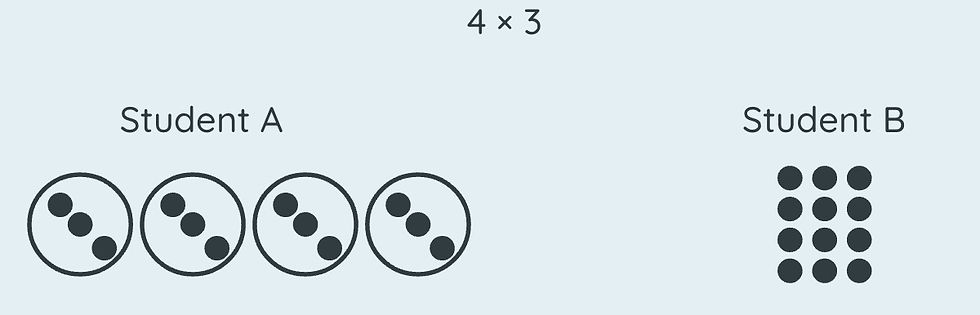
Options of what to compare to enhance learning
Correct examples for two of the same type of problem (enhance problem-solving skills)
Compare correct solutions to two separate problem types (enhance problem-solving skills)
Compare correct and incorrect solutions to the same problem
Same benefits as studying and explaining incorrect worked examples
Comparing two solutions to the same problem helps
Determine which strategy is better
This leads to flexibility in choosing the best strategy for a variety of problems
Helps students consider more than one strategy for solving a problem
To get the most out of this type of worked example, students may need a certain threshold of prior knowledge to really benefit
Concept retention emerged only for high-level learners
Which Type Do I Choose?
Some worked examples lend themselves better to certain problem types than others.
If your goal is procedural fluency, a faded work example might be best
Conclusions
Use worked examples! They should be used a lot more than they are, students benefit more from studying why something does or does not work than from doing endless problems incorrectly
Can be used up to ½ of the time!
They help students develop a deep understanding of why something works instead of just procedures or algorithms that they can then apply to future problems.
Learning from mistakes can help students learn a lot! Studying errors can help students see mistakes as opportunities for learning instead of something to dread
Worked examples help improve student conceptual understanding and procedural skills.
Further Reading Simple Practice Doesn’t Always Make Perfect: Evidence from the Worked Example Effect by Julie L Booth et al

Comments